Assessing Adherent Cell Mechanical Behavior by 6 and 12-Strut Tensegrity Model

Introduction

Most functions of living cells, including cell growth and differentiation, are related to cell shape deformation. Transfiguration of cells is dependent on the cytoskeleton behavior more than any other organelles [1]. In addition to its effect on cell shape, structure and deformation, the cytoskeleton is the main factor of transferring mechanical forces through the cell [2,3]. The cytoskeleton is composed of three main strands of the actin, microtubule and intermediate filaments. Many experiments have proved that actin and intermediate filaments are under tensile forces, while microtubules are suitable to withstand compressive forces [4,5]. To assess the overall behavior of the cytoskeleton, several computational models like pre-tensioned cable network [6] and semi-flexible network [7] have been presented. Most of these models focus only on the tensile elements. One of the computational models is a tensegrity model in which both of the compressive elements and tensile elements are involved.
Tensegrity is a self-balancing structure in which non-connected compact struts (isolated rods) are floating in a continuous elastic environment. Ingber [6] showed that for a living cell, the struts resemble the behavior of the microtubules, and the cables represent actin filaments. Each of the cell components has its own specific role in cell behavior and balance [8]. In tensegrity structure, pretension in actin filaments can be balanced with focal adhesion, that is why tensegrity is not a proper structure to consider non-adherent cells. Because in non-adherent cells there is no focal adhesion to transfer mechanical stress through the membrane [9]. In this study, only adherent cells have been considered. Previous studies applied the structures of different complexities for studying various aspects of the cells’ mechanical behavior [1,10-12]. Prendergast, et al. [13] studied tissue response to mechanical stresses, trying to model a single spread cell adhered to the substrate while influenced by shear stress. He also used 6-strut tensegrity structure for the purpose of modelling cytoskeleton and solid parts for the membrane, nucleus and cytoplasm [13]. Kardas, et al. [14] modelled an osteocyte inside the lacunae.
The cell structure has involved a different type of cell component like cytoskeleton, nucleus, and integrin. Three different models made by fiber elements have been created for presenting actin network, intermediate filaments connected to the nucleus, and microtubules which were connected to the centrosome, while a 30 struts tensegrity has been chosen for modeling the nucleus. They also examined various types of cytoskeleton fibers arrangement in the cell. Their study showed that random arrangement is more consistent with experimental observations [14]. The way of estimating the nucleus by using the tensegrity structure has been recorded by Ingber [15,16]. another research on tensegrity as cell cytoskeleton structure is the Bursa and Fuis study [17]. They presented a FEM model for the eukaryotic cell. Their model involved tensegrity (cytoskeleton), membrane, cytoplasm, and nucleus. They simulated 60 peripheral cables and 30 radial cables, which had the same joint points at the cellular level.
This model is proper to show transition extracellular force to the fiber part of the cell. De Santis, et al. [18] have used the tensegrity structure for investigating cell spreading behavior on the substrate. They used a FEM model of 6-strut tensegrity to find out how the cell feels the surface stiffness. They applied a tensegrity structure with a solid membrane, cytoplasm, and nucleus to a spread cell attached to an elastic substrate. They finally observed that there is a one-to-one relationship between microtubules’ compression and the substrate elasticity [18]. To compare the complexity of the structure, Chen, et al. [19], used 6 and 12-strut to simulate intracellular force distribution and stored energy in cell spreading. They noticed that 12-strut tensegrity has more flexibility to spread. They also showed that during cell spreading, the stored energy in cables increased but microtubules would limit the cell spreading by decreasing energy to zero [19]. Several usages of tensegrity have been found for estimating cellular behavior, but there is a simple question here. Could the same structure be used for different cell phenotypes with different stiffness? How should it be dealt with cell stiffness?
The objective of this study is to assess the role of tensegrity’s structure complexity on adherent cell behavior cell. A vertical deflection was applied to the structure to mimic BioMEM system for measuring cell compliance [20] experiment, and the reaction forces of the structure to this displacement were assessed. Then, the reaction force of this structure vs. the substrate stiffness changes was investigated to study the effect of the substrate stiffness on cellular behavior.

Methods

Two types of cytoskeletal structures based on spherical tensegrity with 6 and 12-strut models are proposed. All the vertices of the tensegrity model are the coupling points of the struts and cables, and all of them are located on the circumference of a sphere. The diameter of the sphere is as equal as cell diameter. The 6-strut tensegrity was produced by three perpendicular hypothetical planes, all of which pass through the center of the sphere. There was a pair of parallel struts on each plane located on both sides of the center of the model, and none of the pairs of struts were parallel to each other. After adjusting the struts in their positions, the end of each strut was connected to the end of the nearest adjacent struts, that is, all the adjacent vertices in the model connected by cable. This connection was made in a manner where each strut is surrounded by four cables. Finally, the 6-strut tensegrity structure was completed by adding 24 cables [21]. The 12-strut tensegrity is a spherical structure, in which four hypothetical planes are overlapped only in one point (the central point of the sphere).
In this structure, there are three struts in each hypothetical plane. The struts are placed together in a triangle-like structure and the head or end of the strut are connected to the nearest adjacent vertices through four cables. The 12-strut tensegrity model is completed by adding 48 cables [21]. In the cytoskeleton, the end of each microtubule which is close to the membrane is connected to the integrin. Integrin is a transversal membrane’s proteins that are contacted to the extracellular environments outside of the cell and transfer all extracellular interactions into the cell. Inside the cell, integrin sends the extracellular forces toward the cytoskeleton and makes the filaments to sense tensile stresses. This initial tensile force is subjected to microtubules compression and causes a forced balance in the whole cytoskeleton. This force balance of filamentary strands eventually leads to balance in the shape and structure of the cell. In fact, the stability of the tensegrity structure is dependent on the pretension of cables. In this simulation, pres-tress of 82kPa for the initial tension of cables and 12.4kPa for compressive prestress of struts were applied [22].
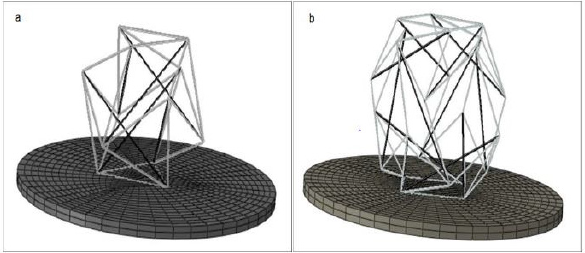
For substrate, an isotropic elastic solid disk was created. Then, in order to connect the structure to the substrate, the tensegrity structure was placed on the substrate in such a way that some of the struts ends touched the substrate. The cell model structure had was created using 6 and 12-strut tensegrity, the cell was places on the substrate so three and four cables were contiguous with the substrate, respectively (Figure 1).

Figure 1: Cell placement on the substrate,
a) 6-strut tensegrity model,
b) 12-strut tensegrity model (elements that are shown in gray and black represent cables and struts, respectively).

Dimensions and Mechanical Properties of Cytoskeleton Components

In the 6-strut tensegrity model, the length of each strut was 14μm, and the distance between two parallel struts was 7μm with a strut cross-section area of 1340 nm2. Young’s modulus and Poisson’s ratio was defined as 1.2GPa and 0.3, respectively [23,24]. Cables are tensile elements with Young’s modulus of 2.6GPa and Poisson’s ratio of 0.3. The cross-sectional area of cables was 570nm2. The substrate was an elastic disk of 1 μm thickness and 30μm diameter. The values of 100 kPa and 0.3 were designated for Young’s modulus and Poisson’s ratio of the substrate, respectively [24].

Loads and Boundary Condition

AFM is a regular and standard experimental method in cell mechanical behavior studies [25]. However, AFM has a sharp point of the contact that may result in the local strain concentration. The alternative method of cell loading using a BioMEM system for measuring cell compliance was suggested [20] that allowed application of the load to the whole upper surface of the cell; thus a similar displacement was applied to all points representing the cell’s upper surface. In order to simulate the BioMEMs load, a downward displacement was applied to the upper vertices of the structure (3 and 4 vertices for 6 and 12-strut tensegrity, respectively). All degrees of freedom for the nodes located at the bottom surface of the disk (substrate) were fixed. In order to attach the tensegrity structure to the substrate, all connected vertices with the disc (located at the end of microtubules) were pinned to the nearest node of the substrate meshes. To study the cytoskeleton behavior, the applied displacement to the upper vertices of the structure (Figure 2) was incremented from 0.1 to 1 μm, and the total reaction force at these nodes was calculated.

Figure 2: Applied displacement and boundary conditions for the substrate and cell.

Solution

Linear momentum conservation equations (Newton’s second law) is the governing equation of this problem in this study. If σij is the Cauchy stress tensor, the stress form of momentum is presented as Eq. (1):

Here, parameters ρ is the density, b is the body force and a is the linear acceleration. For static condition and avoiding the presence of body forces like weight, Eq. (1) will be modified as:

To solve this differential equation with the FEM, ABAQUS (version 6.4) solver was used [26]. ABAQUS generates a system of equations and solves them by calculating the stiffness matrix and the displacements at any nodes.

Meshing

To model the cables and struts, tension only and compression only truss elements (T3D2) were chosen respectively. For meshing the substrate, the 8-node 3D structural element (C3D8R) was. After the mesh convergence 1824 elements were obtained for the substrate. Nonlinear geometry (Nlgeom) option was checked in step modulus to encounter large deformation problem.

Results

Effect of the Variation of Number of The Strut Elements

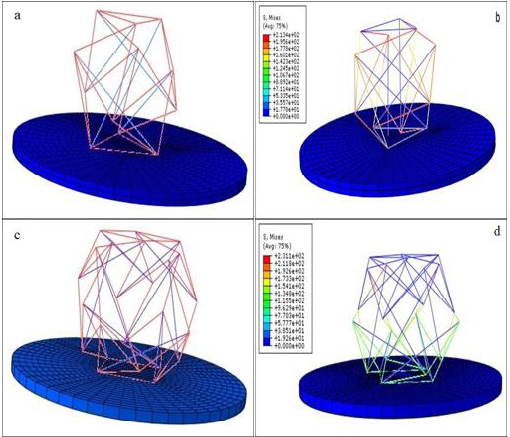
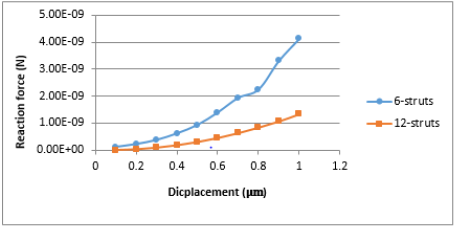
The color representation of the stress magnitudes carried by the struts and cables are shown in Figure 3. The effect of the applied prestresses on the elements for both 6 and 12 strut structures are shown in Figures 3a & 3c, while Figures 3b & 3d shows the stress in the structure components when it is deformed by external load. In the prestressed structures, each element is loaded by a prescribed tensile or compressive stress. The uniformity of the elements colors indicates that each member is under either constant tension (cables) or compression (struts). Analysis of the reaction force vs. deformation shows that increased external displacement leads to increases in the reaction force, as one should expect (Figure 4). The obtained data shows that the 6-strut tensegrity model exhibits larger stiffness compared to a 12-strut. Therefore, in order to model behavior of the cell with higher stiffness, application of the 6-struts tensegrity model may be advantageous.

Figure 3: Strut and cable stresses in the 6 and 12-strut tensegrity structures. Pre-stress only
a) for the 6-struts structure and,
b) 6-struts structure and,
c) for the 12-struts structure, external load
d) 12-struts structure.

Figure 4: Force-deflection diagram for 6 and 12-strut tensegrity structures.

Effect of the Substrate Stiffness

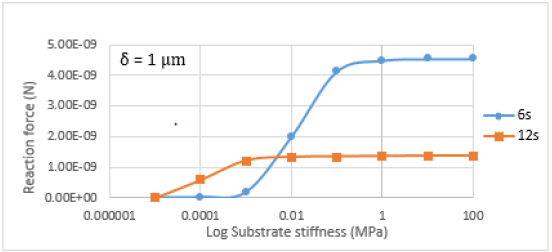
Substrate stiffness is one of the important factors affecting the adherent cell in vitro behavior. Depending on their types, cells are sensitive to a specified range of substrate stiffness. The changes in substrate stiffness cause changes in cytoskeleton balancing forces and modifies cell movement along the substrate [18,27]. In order to evaluate the effect of substrate stiffness on the cytoskeleton, substrate stiffness was changed from 10 Pa to 100 MPa and the tensegrity structure’s reaction force was calculated for 1μm downward displacement. Figure 5 demonstrated the variation of the reaction force due to the change of the substrate stiffness for δ=1 μm. Let us define the area where reaction force is sensitive to the change of the structure’s stiffness as an area of cell sensitivity to the substrate stiffness. This area has a range of 1 to 100 kPa for 6-strut tensegrity. While 12-strut tensegrity does not depend on stiffness greater than 1 kPa.
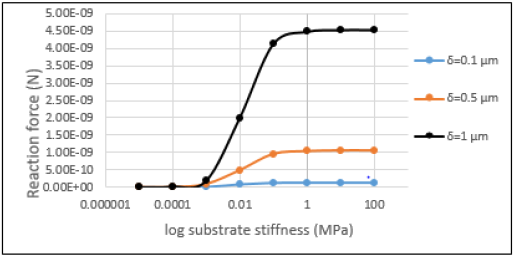
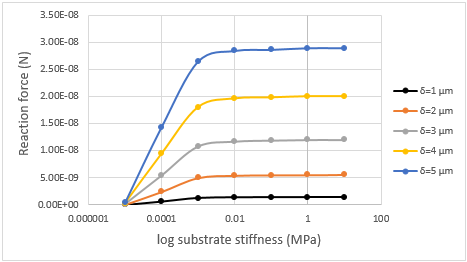
Since the forces of the cytoskeleton are balanced through substrate stiffness, it can be claimed that the 6-strut tensegrity compared to 12-strut structure approximates stiffer cells. So, an increase in the number of struts makes the structure more flexible and more appropriate to estimate softer cells’ modeling. The variation of the reaction force along with the stiffness of the substrate is shown in Figure 6. As demonstrated here, the sensitivity area is independent of the amount of motility, while reaction force increases by a rise in displacement. The reaction force changes against substrate stiffness for the 12-strut structure are shown in Figure 7. In a 6-strut structure, the response force sensitivity increases with increasing displacement, while the sensitivity area is the same and does not change by increasing displacement. By comparing Figures 5 & 6, it is concluded that in the 6-struts structures, the variation of the reaction force is greater than that of the 12-strut structure. This 6-strut tensegrity is more appropriate for modelling the stiffer cell behavior.

Figure 5: The reaction force vs substrate stiffness for the 6 and 12-strut tensegrity models.

Figure 6: The 6-strut reaction force vs applied displacement.

Figure 7: 12-strut reaction Force vssubstrate stiffness in different AFM displacement.

Discussion

Each spherical tensegrity model can be built with the help of a regular polyhedron. Each vertex of the structure is aligned with the polyhedron’s corners located on the surface of the sphere. The obtained results showed that a tensegrity with fewer number of struts creates a stiffer structure. By creating tensegrity structures of the same size, but with increased number of struts, the structure configuration will look more similar to the dome while the length of the struts will decrease, and stiffness of the structure will increase. By reducing the length of the struts, and increasing distance from the center of the sphere, struts have less contribution in tolerating foreign forces, and this makes the structure more flexible. In the area of the cell sensitivity (Figure 5), the balance between cytoskeleton structure and mechanical environment depends on the substrate stiffness. Under the applied external displacement, the shape of the structure will change until the internal forces of the components can withstand the external stimulus. The cytoskeleton attaches to integrin within the membrane by the ends of the microtubules. Hence, integrin could be responsible for microtubule mechanical connection to ECM in biological condition and to the substrate in vitro [28] In vitro conditions the microtubules’ internal forces fluctuate due to the changes in substrate properties. Due to such changes the microtubule internal force will change and, accordingly, the cytoskeleton shape will change to reach another equilibrium configuration.

Statement of Ethics

The study was conducted ethically in accordance with the World Medical Association Declaration of Helsinki. Written informed consent was obtained from the patient for publication of this case report and any accompanying images. A copy of the written consent is available for review by the Editor of this journal.

Conclusion

In this study, two types of tensegrity structure were studied as possible models to approximate the cytoskeleton behavior. Cells were loaded by a uniform displacement applied to the top surface. It is recommended to use a structure with fewer struts to simulate stiffer cell behavior. The cell was attached to the substrate to show in vitro condition. Evaluation of the cell response to alerting substrate mechanical properties (Young’s modulus) showed lower sensitivity range for the structure with more compressive elements. This outcome is compatible with the previous studies and confirms that for simulating softer cells, a tensegrity model with more struts is more suitable.

For more Articles on : https://biomedres01.blogspot.com/