Growth of Phytoplankton
Introduction
Phytoplankton Dynamical System
In the paper of Bernard - Gouze [1] one analyse a model of phytoplankton growth based on the dynamical system
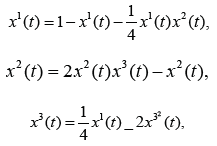
where x1 means the substrate, x2 is the phytoplankton biomass and x3 is the intracellular nutrient per biomass, with the physical domain 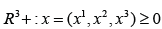 The previous dynΣamical system is non cooperative and has the equilibrium point
The previous dynΣamical system is non cooperative and has the equilibrium point 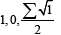 We introduce the phytoplankton vector field
We introduce the phytoplankton vector field  of components
of components 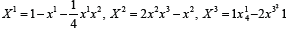 and the maximal field line x = x(t, x0), t ∈ I, which satisfies the initial condition x(t0, x0) = x0. In order to find bounds for substrate, biomass, and intracellular nutrient per biomass, we use the techniques of optimization developed in our papers [2-6].
and the maximal field line x = x(t, x0), t ∈ I, which satisfies the initial condition x(t0, x0) = x0. In order to find bounds for substrate, biomass, and intracellular nutrient per biomass, we use the techniques of optimization developed in our papers [2-6].
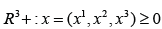 The previous dynΣamical system is non cooperative and has the equilibrium point
The previous dynΣamical system is non cooperative and has the equilibrium point  We introduce the phytoplankton vector field
We introduce the phytoplankton vector field 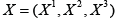 of components
of components 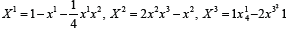 and the maximal field line x = x(t, x0), t ∈ I, which satisfies the initial condition x(t0, x0) = x0. In order to find bounds for substrate, biomass, and intracellular nutrient per biomass, we use the techniques of optimization developed in our papers [2-6].
and the maximal field line x = x(t, x0), t ∈ I, which satisfies the initial condition x(t0, x0) = x0. In order to find bounds for substrate, biomass, and intracellular nutrient per biomass, we use the techniques of optimization developed in our papers [2-6].Bounds for Phytoplankton Substrate
We use the following problem: ftnd max f (x1, x2, x3) = x1 with the restriction x=x(t,x0). We set the critical point condition 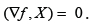 . In this case
. In this case 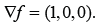 It follows the relation
It follows the relation 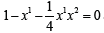 The convenient solution (critical point)
The convenient solution (critical point) 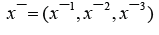 must be
must be
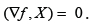 . In this case
. In this case 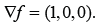 It follows the relation
It follows the relation 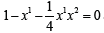 The convenient solution (critical point)
The convenient solution (critical point) 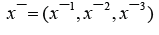 must be
must be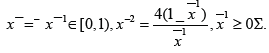
The sufficient condition Hess 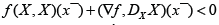 reduces to
reduces to 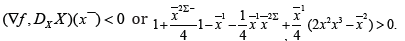
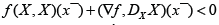 reduces to
reduces to 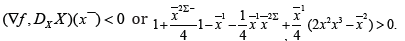
Since at the critical point we have 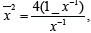 the condition goes to
the condition goes to 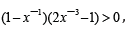 and the convenient condition is
and the convenient condition is 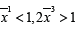 . Theorem 2.1. Suppose that on a evolution line (field line) it exists a point x¯ at which we have
. Theorem 2.1. Suppose that on a evolution line (field line) it exists a point x¯ at which we have
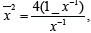 the condition goes to
the condition goes to 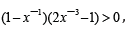 and the convenient condition is
and the convenient condition is 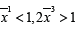 . Theorem 2.1. Suppose that on a evolution line (field line) it exists a point x¯ at which we have
. Theorem 2.1. Suppose that on a evolution line (field line) it exists a point x¯ at which we have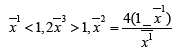
Then the phytoplankton substrate has an upper bound at this point.
Bounds for Phytoplankton Biomass
Let us use the problem: ftnd max 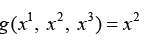 subject to x=x(t,x0).
subject to x=x(t,x0).
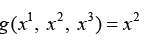 subject to x=x(t,x0).
subject to x=x(t,x0).Growth of phytoplankton: The critical point condition is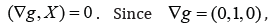 it follows the relation
it follows the relation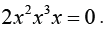 The convenient solution (critical point)
The convenient solution (critical point)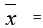
 The sufficient condition Hess
The sufficient condition Hess
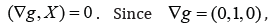 it follows the relation
it follows the relation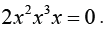 The convenient solution (critical point)
The convenient solution (critical point)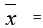
 The sufficient condition Hess
The sufficient condition Hess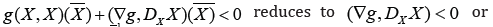
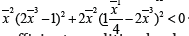 Since at the critical point we have , the sufficient condition leads to
Since at the critical point we have , the sufficient condition leads to 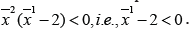
Theorem: Suppose that on a evolution line (field line) it exists a point at which we have
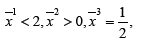
then the x2(t) component of the corresponding field line has an upper bound at this point.
In the direct alternative, we build the composite function g(x(t, x0)). The condition
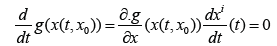
reduces to 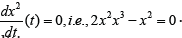 the convenient solution is
the convenient solution is 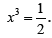 The condition
The condition 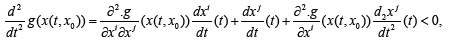 becomes
becomes 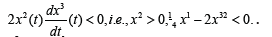 Replacing
Replacing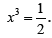 we find x2 > 0, x1 − 2 < 0. The same result is obtained as in the previous method.
we find x2 > 0, x1 − 2 < 0. The same result is obtained as in the previous method.
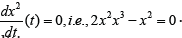 the convenient solution is
the convenient solution is 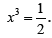 The condition
The condition 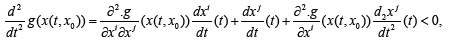 becomes
becomes 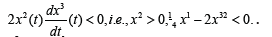 Replacing
Replacing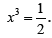 we find x2 > 0, x1 − 2 < 0. The same result is obtained as in the previous method.
we find x2 > 0, x1 − 2 < 0. The same result is obtained as in the previous method.Bounds for Intracellular Nutrient Per Biomass
Now the helping problem is: compute max 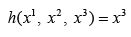 with the restriction
with the restriction 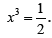
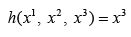 with the restriction
with the restriction 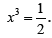
The critical point condition is 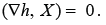 Since Δh = ∇h = (0,0,1), it follows the relation
Since Δh = ∇h = (0,0,1), it follows the relation 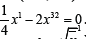 The critical point
The critical point 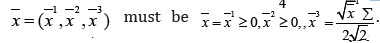 The sufficient condition Hess
The sufficient condition Hess 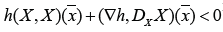 reduces to
reduces to 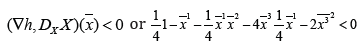
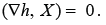 Since Δh = ∇h = (0,0,1), it follows the relation
Since Δh = ∇h = (0,0,1), it follows the relation 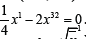 The critical point
The critical point 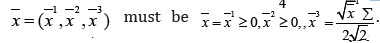 The sufficient condition Hess
The sufficient condition Hess 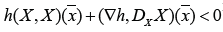 reduces to
reduces to 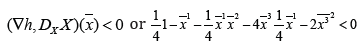
Since at the critical point we have 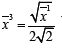 the condition goes to
the condition goes to
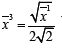 the condition goes to
the condition goes to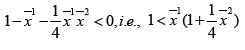
Theorem: Suppose that on a evolution line (field line) it exists a point x at which we have 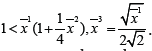 Then the intracellular nutrient per biomass has an upper bound at this point.
Then the intracellular nutrient per biomass has an upper bound at this point.
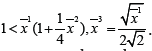 Then the intracellular nutrient per biomass has an upper bound at this point.
Then the intracellular nutrient per biomass has an upper bound at this point.Hypertension and Its Treatment in the Elderly-https://biomedres01.blogspot.com/2020/12/hypertension-and-its-treatment-in.html
More BJSTR Articles : https://biomedres01.blogspot.com
No comments:
Post a Comment
Note: Only a member of this blog may post a comment.